Most useful processes for Truss Analysis

A truss is just like a structure that includes a number of bars pin attached at their ends to develop a solid framework. It is usually taken into consideration that loads and reactions are implemented to the truss only at the joints. A truss is usually formed with triangular elements containing the bars on the upper chord under compression and those along the lower chord under tension. Trusses are mostly utilized for bridges, long span roofs, electric tower, and space structures.
Trusses are statically determinate when the complete bar forces are set from the equations of statics alone. If not the truss is statically indeterminate. A truss appears to be statically (externally) determinate or indeterminate pertaining to the reactions (over 3 or 6 reactions in 2D or 3D problems correspondingly).
In order to analyze truss, it is anticipated that:
Bars are pin-connected.
Joints are frictionless hinges.
Loads are used at the joints only.
Stress in each member is persistent along its length.
The truss analysis is undertaken to define the reactions and member forces. The procedures used for accomplishing the analysis by applying the equations of equilibrium and by taking into account only parts of the structure via analyzing its free body diagram to work out the unknowns.
1.Method of Joints for Truss Analysis
It is supposed that all members remain in tension reaction. A tension member experiences pull forces at both ends of the bar and generally described with positive (+ve) sign. If a member encounters a push force at both ends, then the bar is pointed out to remain in compression mode and described with negative (-ve) sign.
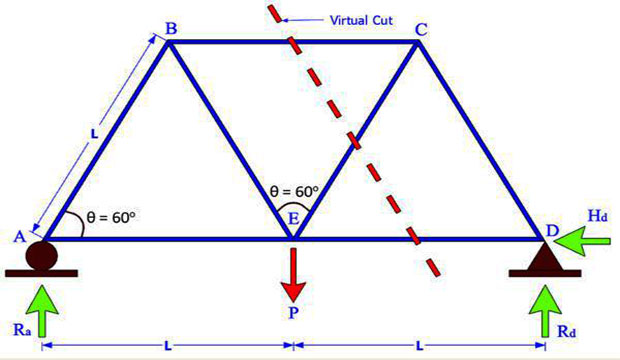
Under the joints method, a virtual cut is prepared around a joint and the cut portion is segregated as a Free Body Diagram (FBD). Applying the equilibrium equations of ? Fx = 0 and ? Fy = 0, the unidentified member forces are determined. It is supposed that all members are combined jointly in the form of an ideal pin, and that all forces are in tension (+ve reactions).
An frictional section may be entirely passed around a joint in a truss. The joint turns out to be a free body in equilibrium under the forces functional to it. The equations ? H = 0 and ? V = 0 will be functional to the joint to define the unidentified forces in members meeting there. It is clear that no more than two unknowns are set at a joint with these two equations.
To read the complete article, go through the following link
www.engineersdaily.com